Armstrong number
In number theory, a narcissistic number or pluperfect digital invariant (PPDI) or Armstrong number[4] is a number that in a given base is the sum of its own digits to the power of the number of digits.
To put it algebraically, let  be an integer with representation dkdk − 1...d1 in base-b notation. If
be an integer with representation dkdk − 1...d1 in base-b notation. If  then n is a narcisstic number. For example, the decimal (Base 10) number 153 has three digits and is a narcissistic number, because:
then n is a narcisstic number. For example, the decimal (Base 10) number 153 has three digits and is a narcissistic number, because:
If the constraint that the power must equal the number of digits is dropped, so that for some m it happens that  then n is called a perfect digital invariant or PDI.[5][2] For example, the decimal number 4150 has four digits and is the sum of the fifth powers of its digits
then n is called a perfect digital invariant or PDI.[5][2] For example, the decimal number 4150 has four digits and is the sum of the fifth powers of its digits
so it is a perfect digital invariant but not a narcissistic number.
In "A Mathematician's Apology", G. H. Hardy wrote:
- There are just four numbers, after unity, which are the sums of the cubes of their digits:
- 153 = 13 + 53 + 33
- 370 = 33 + 73 + 03
- 371 = 33 + 73 + 13
- 407 = 43 + 03 + 73.
- These are odd facts, very suitable for puzzle columns and likely to amuse amateurs, but there is nothing in them which appeals to the mathematician.
Narcissistic numbers in various bases
The sequence of "base 10" narcissistic numbers starts: 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 153, 370, 371, 407, 1634, 8208, 9474 ... (sequence A005188 in OEIS)
The sequence of "base 3" narcissistic numbers starts: 0,1,2,12,122
The sequence of "base 4" narcissistic numbers starts: 0,1,2,3,313
The number of narcissistic numbers in a given base is finite, since the maximum possible sum of the kth powers of a k digit number in base b is
and if k is large enough then
in which case no base b narcissistic number can have k or more digits.
There are 88 narcissistic numbers in base 10, of which the largest is
- 115,132,219,018,763,992,565,095,597,973,971,522,401
Unlike narcissistic numbers, no upper bound can be determined for the size of PDIs in a given base, and it is not currently known whether or not the number of PDIs for an arbitrary base is finite or infinite.
Related concepts
The term "narcissistic number" is sometimes used in a wider sense to mean a number that is equal to any mathematical manipulation of its own digits. With this wider definition narcisstic numbers include:
-
- Constant base numbers :
 for some m.
for some m.
- Constant base numbers :
where di are the digits of n in some base.
Program CODE for FORTRAN
PROGRAM Armstrong Number
IMPLICIT NONE
INTEGER :: a, b, c ! the three digits
INTEGER :: abc, a3b3c3 ! the number and its cubic sum
INTEGER :: Count ! a counter
Count = 0
DO a = 0, 9 ! for the left most digit
DO b = 0, 9 ! for the middle digit
DO c = 0, 9 ! for the right most digit
abc = a*100 + b*10 + c ! the number
a3b3c3 = a**3 + b**3 + c**3 ! the sum of cubes
IF (abc == a3b3c3) THEN ! if they are equal
Count = Count + 1 ! count and display it
WRITE(*,*) 'Armstrong number ', Count, ': ', abc
END IF
END DO
END DO
END DO
END PROGRAM ArmstrongNumber
Perfect number
In mathematics, a perfect number is defined as a positive integer which is the sum of its proper positive divisors, that is, the sum of the positive divisors excluding the number itself. Equivalently, a perfect number is a number that is half the sum of all of its positive divisors (including itself), or σ(n) = 2n.
The first perfect number is 6, because 1, 2, and 3 are its proper positive divisors, and 1 + 2 + 3 = 6. Equivalently, the number 6 is equal to half the sum of all its positive divisors: ( 1 + 2 + 3 + 6 ) / 2 = 6.
The next perfect number is 28 = 1 + 2 + 4 + 7 + 14. This is followed by the perfect numbers 496 and 8128Even perfect numbers
Euclid discovered that the first four perfect numbers are generated by the formula 2n−1(2n − 1):
- for n = 2: 21(22 − 1) = 6
- for n = 3: 22(23 − 1) = 28
- for n = 5: 24(25 − 1) = 496
- for n = 7: 26(27 − 1) = 8128.
Noticing that 2n − 1 is a prime number in each instance, Euclid proved that the formula 2n−1(2n − 1) gives an even perfect number whenever 2n − 1 is prime (Euclid, Prop. IX.36).
Ancient mathematicians made many assumptions about perfect numbers based on the four they knew, but most of those assumptions would later prove to be incorrect. One of these assumptions was that since 2, 3, 5, and 7 are precisely the first four primes, the fifth perfect number would be obtained when n = 11, the fifth prime. However, 211 − 1 = 2047 = 23 × 89 is not prime and therefore n = 11 does not yield a perfect number. Two other wrong assumptions were:
- The fifth perfect number would have five digits in base 10 since the first four had 1, 2, 3, and 4 digits respectively.
- The perfect numbers' final digits would go 6, 8, 6, 8, alternately.
The fifth perfect number (33550336 = 212(213 − 1)) has 8 digits, thus refuting the first assumption. For the second assumption, the fifth perfect number indeed ends with a 6. However, the sixth (8 589 869 056) also ends in a 6. It is straightforward to show that the last digit of any even perfect number must be 6 or 8.
In order for 2n − 1 to be prime, it is necessary but not sufficient that n should be prime. Prime numbers of the form 2n − 1 are known as Mersenne primes, after the seventeenth-century monk Marin Mersenne, who studied number theory and perfect numbers.
Over a millennium after Euclid, Ibn al-Haytham (Alhazen) circa 1000 AD realized that every even perfect number is of the form 2n−1(2n − 1) where 2n − 1 is prime, but he was not able to prove this result.It was not until the 18th century that Leonhard Euler proved that the formula 2n−1(2n − 1) will yield all the even perfect numbers. Thus, there is a concrete one-to-one association between even perfect numbers and Mersenne primes. This result is often referred to as the Euclid-Euler Theorem. As of September 2008, only 46 Mersenne primes are known,[2] which means there are 46 perfect numbers known, the largest being 243,112,608 × (243,112,609 − 1) with 25,956,377 digits.
The first 39 even perfect numbers are 2n−1(2n − 1) for
- n = 2, 3, 5, 7, 13, 17, 19, 31, 61, 89, 107, 127, 521, 607, 1279, 2203, 2281, 3217, 4253, 4423, 9689, 9941, 11213, 19937, 21701, 23209, 44497, 86243, 110503, 132049, 216091, 756839, 859433, 1257787, 1398269, 2976221, 3021377, 6972593, 13466917 (sequence A000043 in OEIS).
The other 7 known are for n = 20996011, 24036583, 25964951, 30402457, 32582657, 37156667, 43112609. It is not known whether there are others between them.
It is still uncertain whether there are infinitely many Mersenne primes and perfect numbers. The search for new Mersenne primes is the goal of the GIMPS distributed computing project.
Since any even perfect number has the form 2n−1(2n − 1), it is a triangular number, and, like all triangular numbers, it is the sum of all natural numbers up to a certain point; in this case: 2n − 1. Furthermore, any even perfect number except the first one is the sum of the first 2(n−1)/2 odd cubes:
Even perfect numbers (except 6) give remainder 1 when divided by 9. This can be reformulated as follows. Adding the digits of any even perfect number (except 6), then adding the digits of the resulting number, and repeating this process until a single digit is obtained — the resulting number is called the digital root — produces the number 1. For example, the digital root of 8128 = 1, since 8 + 1 + 2 + 8 = 19, 1 + 9 = 10, and 1 + 0 = 1.
Odd perfect numbers
It is unknown whether there are any odd perfect numbers. Various results have been obtained, but none that has helped to locate one or otherwise resolve the question of their existence. Carl Pomerance has presented a heuristic argument which suggests that no odd perfect numbers exist. Also, it has been conjectured that there are no odd Ore's harmonic numbers (except for 1). If true, this would imply that there are no odd perfect numbers.
Any odd perfect number N must satisfy the following conditions:
-
- where:
- The largest prime factor of N is greater than 108 (Takeshi Goto and Yasuo Ohno, 2006).
- The second largest prime factor is greater than 104, and the third largest prime factor is greater than 100 (Iannucci 1999, 2000).
- N has at least 75 prime factors and at least 9 distinct prime factors. If 3 is not one of the factors of N, then N has at least 12 distinct prime factors (Nielsen 2006; Kevin Hare 2005).
- When ei ≤ 2 for every i
- The smallest prime factor of N is at least 739 (Cohen 1987).
- α ≡ 1 (mod 12) or α ≡ 9 (mod 12) (McDaniel 1970).
In 1888, Sylvester stated:
...a prolonged meditation on the subject has satisfied me that the existence of any one such [odd perfect number] — its escape, so to say, from the complex web of conditions which hem it in on all sides — would be little short of a miracle.
Minor results
All even perfect numbers have a very precise form; odd perfect numbers are rare, if indeed they do exist. There are a number of results on perfect numbers that are actually quite easy to prove but nevertheless superficially impressive; some of them also come under Richard Guy's strong law of small numbers:
- An odd perfect number is not divisible by 105 (Kühnel 1949).
- Every odd perfect number is of the form 12m + 1 or 36m + 9 (Touchard 1953; Holdener 2002).
- The only even perfect number of the form x3 + 1 is 28 (Makowski 1962).
- A Fermat number cannot be a perfect number (Luca 2000).
- The reciprocals of the divisors of a perfect number N must add up to 2:
- For 6, we have 1 / 6 + 1 / 3 + 1 / 2 + 1 / 1 = 2;
- For 28, we have 1 / 28 + 1 / 14 + 1 / 7 + 1 / 4 + 1 / 2 + 1 / 1 = 2, etc.
- The number of divisors of a perfect number (whether even or odd) must be even, since N cannot be a perfect square.
- From these two results it follows that every perfect number is an Ore's harmonic number.
- The even perfect numbers are not trapezoidal numbers; that is, they cannot be represented as the difference of two positive triangular numbers. There are only three types of non-trapezoidal numbers: even perfect numbers, powers of two, and a class of numbers formed from Fermat primes in a similar way to the construction of even perfect numbers from Mersenne primes










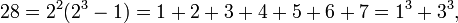
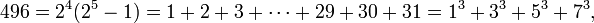
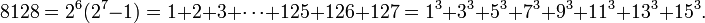

 > 1020 for some j (Cohen 1987).
> 1020 for some j (Cohen 1987). (Nielsen 2003).
(Nielsen 2003).

No comments:
Post a Comment